Is there really a conflict between General Relativity and Quantum Mechanics?

The problem of combining General Relativity with Quantum Mechanics in a self-consistent theory is so difficult that it has defied the best minds in physics for 75 years. But this may not be necessary. We don’t actually know that General Relativity is correct.

You have probably heard that the greatest problem confronting theoretical physics is that we have two incompatible theories. General Relativity is Einstein’s theory of gravity, and it applies in the realm of astronomy, where it has been validated. Quantum Mechanics is a theory of atoms and subatomic particles, and it has been validated in the realm of the very small. The problem is that these two theories both work well, but they are incompatible. Einstein spent the last years of his life trying to combine them, and the world’s smartest physicists have tried ever since without success.

The natural thing to do would be to try experiments with quantum gravity, and use the results to home in on a theory. But no such experiments are feasible. The world’s biggest particle accelerator can reach energies of trillions of electron volts (10^12 eV). Quantum gravity effects are predicted to be discernable at about 10^28 eV. We would need a particle accelerator that is a million billion times bigger than what we’ve got. Of course, we have no idea how to construct such an accelerator, and if we built it using present technology, it would be the size of our galaxy.

So, we’re at a loss to reconcile these two great theories, but we’re not at a loss to explain anything that we can actually observe. Our reason for believing that these two theories ought to be compatible comes from a faith in the Zeroth Law of Science, taken literally. GR and QM must be part of some self-consistent Grand Unified Theory because we have faith that the universe is built on mathematical foundations that are absolutely consistent, and that hold everywhere and forever.

Fifty years ago at Harvard

I was an undergraduate physics major in 1969, in my senior year, taking courses that were over my head because I was ambitious. I was privileged and cursed to receive my introduction to quantum mechanics from Julian Schwinger, a Nobel laureate, and co-inventor of quantum field theory. He left my head spinning three mornings a week.

At the time, there were three classic tests of GR.

1. First, light from a star bends as it passes by the sun. In fact, it bends twice as much as if you just used a naïve model of light as a particle (photon). Of course, you normally can’t photograph a star that’s right next to the sun, but during an eclipse, the sky becomes dark enough that stars right next to the sun become visible. In 1919, Arthur Edington traveled to West Africa explicitly to be in the path of a total eclipse, where he could take a photograph that tested Einstein’s new General Theory of Relativity. The result vindicated Einstein, and the world applauded.
2. Second, the orbits of all planets are not exactly circular. According to Newtonian gravity, the orbit should be an ellipse, fixed in space. But with Einstein’s corrections, the ellipse itself rotates so that the point of the ellipse moves a little further around the sun with each pass through the orbit.  Mercury moves through the orbit in 88 days, but the orbit takes 23,000 years to complete a rotation around the sun. This is called “precession of the perihelion”, and Einstein’s prediction for the rate of precession is thought to be only part of the story. The precession is also driven in the same direction by the sun’s shape, which is slightly oblate, not exactly spherical. It’s far the most complicated calculation of the three, but results are said to vindicate Einstein.

   

3. Third is a gravitational redshift. Light that is rising out of a gravitational field loses energy and becomes redder as a result. If you shine a laser straight up from the first floor of the Physics Dept building at Harvard and intercept the light on the fourth floor with an instrument very sensitive to wavelength, you will find that the wavelength is very slightly longer (the color is very slightly redder) on the 4th floor than it was on the 1st floor. This experiment won a Nobel prize for my my Freshman physics professor, Robert Pound.

All the physics students in Dr Schwinger’s class knew of these three tests. They’re legendary. Imagine our amazement when Dr Schwinger spent three lectures calculating predictions for these three experiments from his own quantum theory of the graviton.

What could this mean? Where’s the conflict between QM and GR? Where’s the problem that the best minds in physics have been obsessing over for the last 75 years?

Answer: For situations that are “close to” Newtonian, GR and QM give the same answer.

Gravity is so weak that the only measurements that we can make are far less precise than measurements in chemistry or electromagnetism or nuclear physics. In most everyday and astronomical applications, the predictions of GR and Newtonian gravity are so close that we can’t tell them apart. And even in the case of these three classic “tests of general relativity”, the difference between GR and Newtonian is tiny. In such cases, GR predicts the same size departure from Newtonian as QM, so in practice the QM theory is perfectly adequate, and we don’t really need GR.

It’s only in the vicinity of a black hole or a neutron star that the difference between GR and Newtonian physics becomes sizable. We certainly do see neutron stars and black holes in the sky, but we can’t use them to verify the GR predictions because we don’t know precisely what we are looking at. We don’t have an independent way to measure the mass of the NS or BH. So, in practice, what astronomers do is to assume that GR is correct and calculate backwards from their observations to make inferences about the BH or the NS.

A calculation trick well-known to physicists

There’s a technique from physics, as old as Newton, by which you can frequently solve a difficult problem approximately. It’s called ‘perturbation theory.’

1. Find a simple version of the same problem
2. Solve the simple version
3. Solve an equation for how much the answer changes when you move away from the simple problem in the direction of the difficult problem
4. Determine by how much your parameters differ from the simple version and use #3 to move along a straight line away from the simple answer by the appropriate amount.
5. If you want a better answer you can repeat the process in #3 and analyze the correction to your correction. This is like moving away from the simple solution along a parabola instead of a straight line.

In the case of Newtonian gravity and Einstein’s GR, the Newtonian answer is easy to calculate, and the GR calculation is usually very difficult — way beyond computation for general cases with odd geometries. The Newtonian answer is the one you would get if you were very far away from a gravitating star or planet.

How big is the difference between the (simple) Newtonian answer and the (hard to calculate) GR answer? The answer is that it is proportional to the ratio of the radius of a hypothetical black hole to the radius at which you’re working.

For example, think about light passing by the sun for Eddington’s 1919 test of GR. If the sun were a black hole, it would have a radius of about 3 Km. The sun’s actual radius, where the starlight passes by the sun during an eclipse, is 700,000 Km. This tells you that the answer from Newtonian gravity and the answer from GR differ by about 3:700,000 or one part in 200,000.

The punch line, the point that I am aiming for, is that all three of the observed confirmations of Einstein’s General Relativity are like this. The differences from Newtonian gravity are very tiny, and it takes special circumstances and delicate measurements to distinguish the relativistic correction to Newtonian gravity.

So all the fuss about needing a way to reconcile quantum field theory with GR is just theoretical. There is no conflict in practice for any quantities that we are able to observe or measure.

What about other, more recent applications of GR?

Certainly there have been other applications of GR since I went to school in the 1960s. Gravitational waves have been observed, but we don’t know where they came from, so can’t use them to distinguish different theories of gravity.

GR is used to make cosmological calculations, for example the age of our universe and the evolution of the universe since the Big Bang. But these calculations assume that GR is true. They are not a test of whether GR is true.

Hawking radiation is a result of GR in the quantum realm, but once again, calculations of Hawking radiation are rooted in theory. Hawking radiation has never been observed in practice.

Gravitational lensing is another GR effect which is used to interpret observations in the sky. When we see a distant galaxy behind a nearby galaxy, sometimes the image is fractured and we see multiple copies of the rear galaxy on opposite sides of the galaxy in front. Or the image of the rear galaxy can be spread out to appear as a ring around the front galaxy.

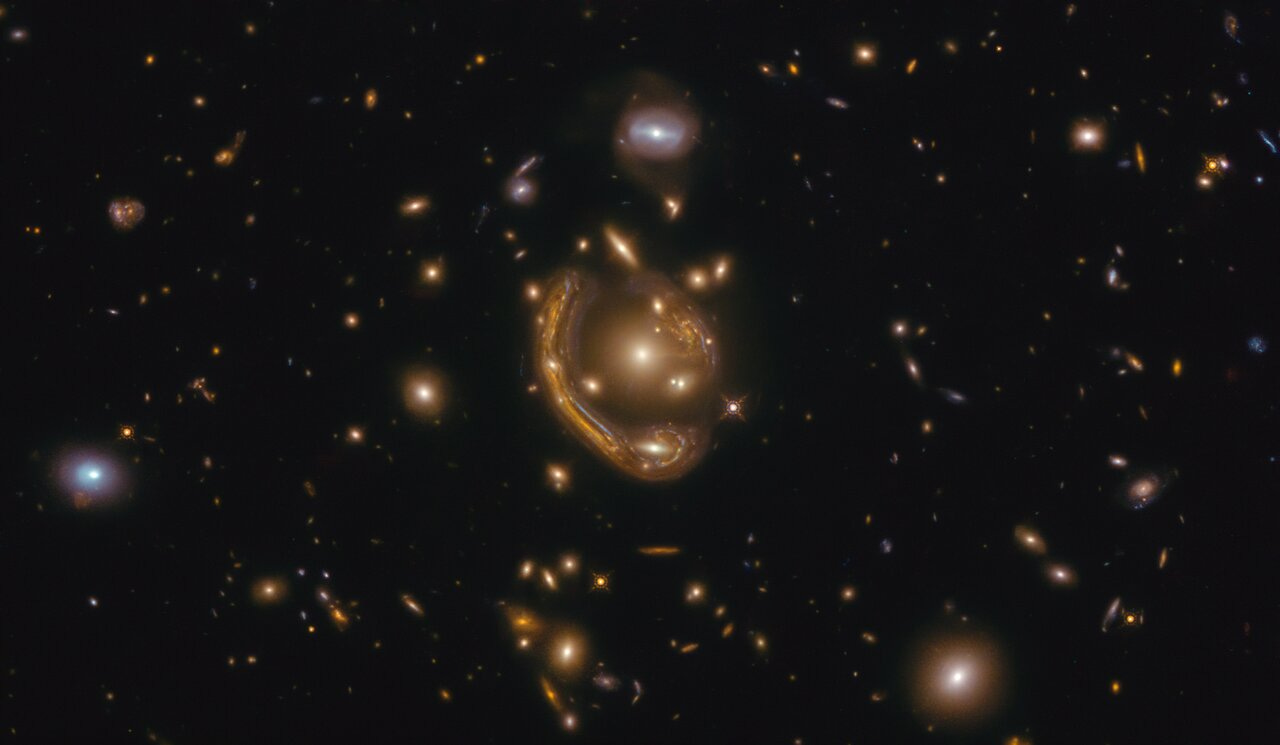
But since we don’t know the mass or position of the close galaxy, no quantitative conclusions can be drawn from gravitational lens observations.

The most stringent test by far is the Global Positioning System, GPS, that we use when we’re driving, and that everyone from pilots to backpackers counts on as well. The GPS system works by comparing the time that it takes a radio signal to reach you from several different satellites. Each satellite is broadcasting the exact time, accurate to a billionth of a second, and the GPS chip in your cell phone picks up three or more time readings that differ from one another very slightly because of the time that it takes the signal to reach you from each of them. It is from these differences that the chip calculates how far you are from each of the satellites, and from this can calculate where you are on the earth’s surface.

The clocks on these satellites run slightly faster than our clocks on earth because the earth’s gravitational field is weaker up there. The difference is very tiny, but large enough that it has to be factored into the calculation in order for the chip to render your position accurately.

This is a GR correction to Newtonian gravity, and it is the best indication that we have that GR actually works — better than the Edington eclipse measurement or the Pound-Rebka experiment or the precession of Mercury. But still, the GR correction is very small, and so it remains in the regime where quantum field theory and GR give the same answer.

Conclusion: As far as we know, quantum field theory of gravitons gives the right answer for everything about gravity that we can actually measure. We’ve tried like the devil to reconcile GR with QFT, without success so far. But it may be that we don’t need to reconcile them. It may be that QFT is all we need.

Physicists love GR because it is built on an elegant mathematical foundation. Simple ideas generate immensely complex equations, and those equations seem to work. This is a physicist’s idea of heaven.

Einstein was interviewed after Eddington verified his General Relativity in 1919. Reportedly, when asked what his reaction would have been if Eddington’s measurements had not supported his predictions, Einstein replied:

“To tell you the truth, I would have been sorry for the dear Lord—the theory is correct.”

Einstein’s General Relativity is the most beautiful theory that anyone has ever come up with, but that doesn’t oblige Nature to live by Einstein’s rules.